www.entitymodelling.org - entity modelling introduced from first principles - relational database design theory and practice - dependent type theory
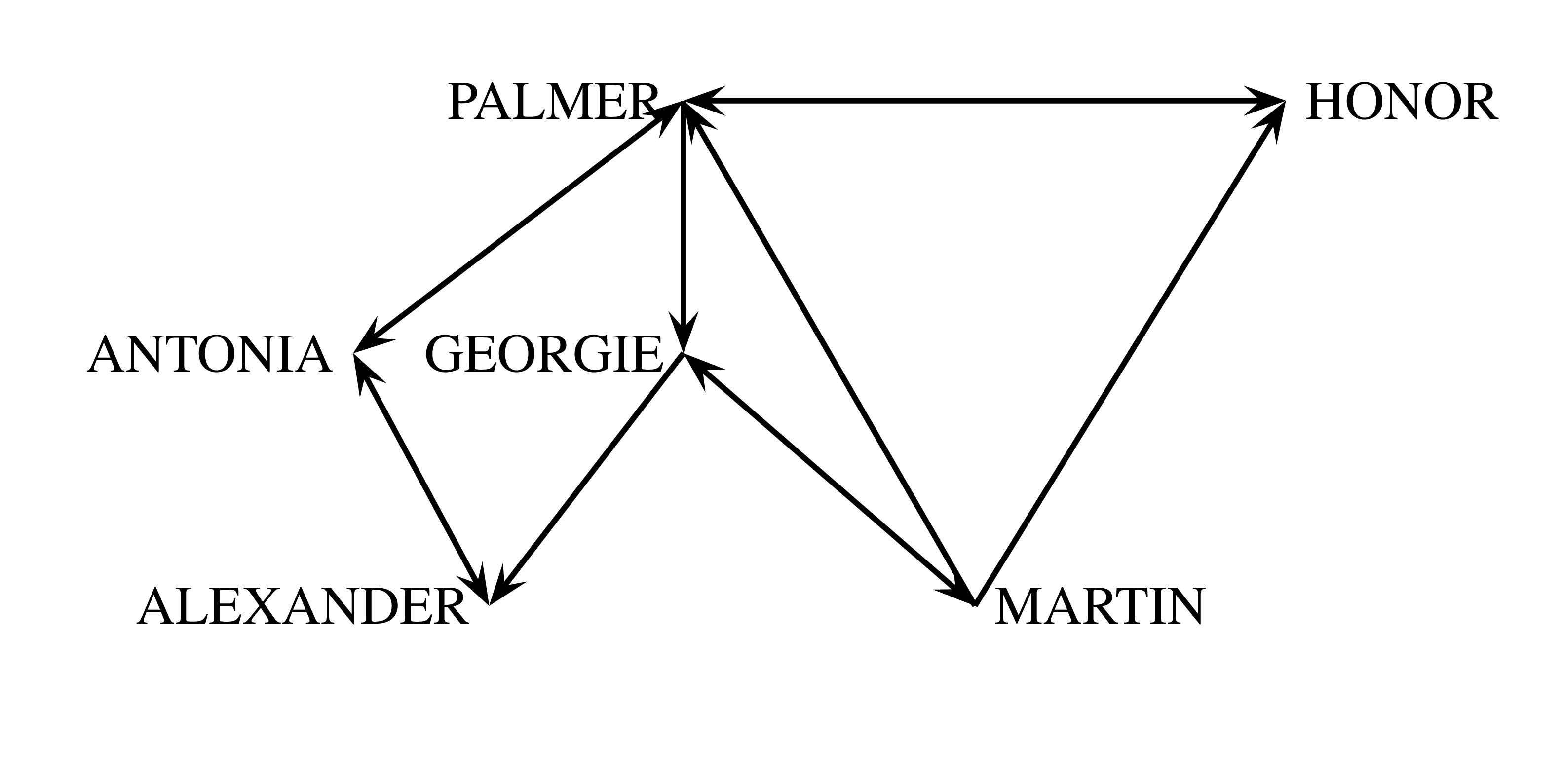
Information Structure Choices
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
||||
|
|
|
|
||||
|
|
|
|
||||
|
|
||||||
|
|
|
|
|
|
||
|
|
|
|
www.entitymodelling.org - entity modelling introduced from first principles - relational database design theory and practice - dependent type theory
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|

|
|
|
|
|
|
|
|
|
|
|
|
||||
|
|
|
|
||||
|
|
|
|
||||
|
|
||||||
|
|
|
|
|
|
||
|
|
|
|